Accelerating Sins - Crank velocity variability

A few days ago I posted this item about the nature of how we apply power while pedalling and what impact this has on the variability in crank rotational velocity during steady state cycling, i.e. cycling along at a steady speed and power.

Part of the reason for examining this, other than for the sheer geek-out fun of it, was to assess whether the variability in crank velocity would violate a key assumption made by most crank/pedal based power meters used to calculate power, that assumption being crank rotational velocity doesn't vary during a pedal stroke, or at least not enough to impact accuracy.

Given the highly variable pulsing sinusoidal-like manner in which power is applied during a pedal stroke (something that has been consistently shown by published scientific measurements of pedal forces since the late 19th Century), then clearly some crank rotational velocity variation during a pedal stroke will exist. However due to the inertia of the bike + rider system, these variations are not typically large if you are using circular chainrings. It's a bit like how a flywheel is used to smooth out the power delivery of an internal combustion or a steam engine.

After discussing a little empirical knowledge and published research, I then examined a few scenarios with a modelling technique known as forward integration.

The outcome of that was, in summary; during steady state cycling at regular riding speeds and power outputs whilst riding outdoors, provided the chain ring is circular, then the amount of crank velocity variation is:
- mostly a function of the inertial load of the system,
- typically pretty small, and
- insufficient to cause concern for power meter accuracy.

However, as crank inertial load reduces significantly (e.g. at low speeds and high power outputs, or when riding on a very low inertia inddor trainer or one with unrealistic resistance) the level of crank rotational velocity variation may begin to introduce some small level of inaccuracy in reported power.

The issue of chain ring shape and the resulting increased variability in crank rotational velocity and associated error in power measurement it introduces to crank/pedal based power meters has been discussed by others, including on Tom Anhalt's blog and Dan Connelly's blog, so I won't go through that here.

OK, that's for steady state cycling. What happens when we are accelerating?

Obviously if we are accelerating, then the crank's rotational velocity must be changing as well. Not only with the trend of overall increase in speed of the rider, but also it will vary up and down about that general increase in speed trend.

So just how much variability in crank rotational velocity is there when accelerating?

I know there are some labs that have actually measured this stuff, but for now I don't have access to such data, so I thought I'd do some more modelling using first order approximations as described in my post the other day, and apply it to an acceleration scenario I have previously modelled.

In December 2014 I wrote Sum of the Parts II, which used similar modelling to compare accelerations with each of two wheel-sets, one that was lighter and less aerodynamic with another that was heavier but more aerodynamic. In that case the aero wheels win just about all the time.

But it had me thinking, as well as another anonymous person who commented on the Sins of Crank Velocity post, what does the bike speed and crank velocity variation look like when accelerating?

So, I thought I'd have a go at modelling it. Great. Now things start to get a little complicated.

First some assumptions:

Assumption 1. 
I'm going to use the power output profile as described in The Sum of the Parts II blog item for a 10-second sprint from a start speed of 30km/h. Here's an image which shows the power curve and speed line that results. It's ~1000W on average but with a power output curve as shown by the yellow dashed line below:

Power starts at 250W, rises quickly to ~ 1250W and then fades through the remainder of the sprint. The rider we can see accelerates from 30km/h to 51km/h. That's a slightly faster end speed than the previous blog post showed, mainly because I'm using a slightly lower value for assumed CdA (aero drag coefficient) in this model (as that's what I used in the crank velocity variation model I highlighted on 1 January).

Given the way power is actually applied in a pulse-like manner, then the real power curve is not as smooth as shown. This is really just a plot of the overall trend in power over that 10-seconds.

Assumption 2. 
No gear changes during the acceleration. I'll assume a 53x15 gear, which is a gear ratio of 7.40 metres. For the track riders out there, that just a bit less than 50x14 gear (7.48m).

Assumption 3.
That power application during a pedal stroke follows a sinusoidal-like pattern but in this case with an ever decreasing period between crank rotations, IOW the cadence is constantly increasing through the acceleration in line with the bike's speed increase. As discussed in the previous item, this is a first order approximation, and the torque profile while accelerating hard will be different to that when riding steady state. We tend to engage our leg muscles differently and also use more upper body musculature in the action. Even so I think using a sinusoidal-like power curve is still a good first order approximation.

Assumption 4.
The model starts with the crank vertical and pedal at top dead centre, power drops to zero watts momentarily each time the crank is in this position - and the left and right legs can apply power symmetrically. Like I said, it's not intended to be a perfect model - just a first order approximation.


Now solving the problem of generating this variable power curve gets a little complicated, and I'm not going to attempt to describe how I did it, but let's just say that it required an iterative process.

Here are some plots to show what's going on. This is the power curve once I overlay a sinusoidal function onto it. It's not a standard sine wave, since the period for each crank rotation reduces as the sprint progresses, and the amplitude varies along with the general power output trend. As always, click on the image to see a larger version.

The basic principle of the peak power being double the average power for the pedal stroke is in line with expected torque/power profiles. As you can see the instantaneous peak power rises to a maximum of nearly 2500W and oscillates between the power peak attained during each down-stroke, and zero watts as the crank arm passes through top dead centre.

If you look at the two red arrows drawn on the bottom axis, you'll see each marks the time taken for the first and the final complete pedal stroke during the 10-second sprint. This is to highlight the ever reducing time period between pedal strokes as cadence increases along the way.

The first full pedal stroke takes 0.87 seconds (69rpm), and the final one 0.53 seconds (114rpm).

Now to overlay the resulting speed curves:

The above plot show 4 lines:

• The smoothed power trend line (dashed yellow)
• The instantaneously variable power line (unbroken yellow) as applied to the cranks
• The smoothed speed trend line (dashed blue)
• The instantaneous speed line (unbroken blue) resulting from the application of variable power 

Here it is without the instantaneously variable power line so you can more easily view the resulting speed plot:

You can see that the actual speed curve oscillates about the general trend line, and that it varies more at the start of the sprint than it does at the end.

Let's zoom in to see this a little more closely. Here's the first 2.5 seconds, with the speed axis zoomed in some more to amplify the variation:

Power rises and falls rapidly, and reaches an maximum instantaneous peak of just under 2500W (actual peak occurs at 2.55 seconds). Thereafter power plateaus and fades.

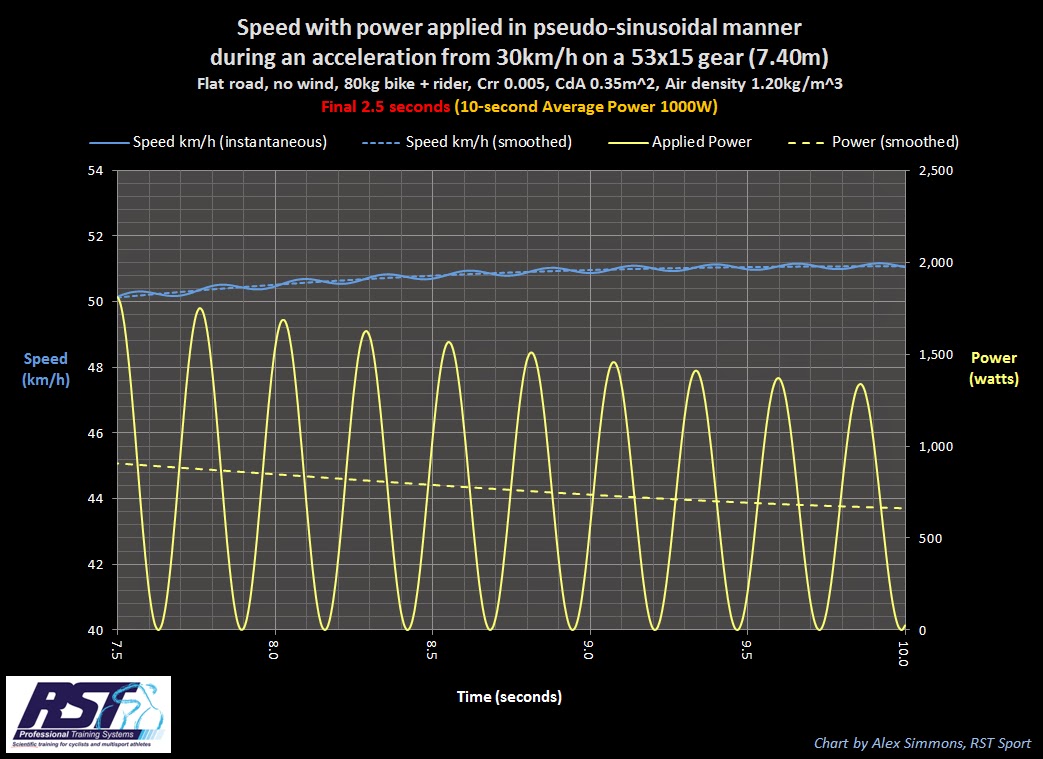
Here are the final 2.5 seconds of the acceleration with the same level of speed scale zoom:

Power in the final 2.5 seconds fades to a trend average of ~660W at the end of the 10-second acceleration

If you look at the speed plots in each, it's pretty clear the level of speed variation, and hence crank rotational velocity variation, is much greater during the early phase of the sprint when speed is lower and power is higher, and the variability reduces as speed rises towards the maximum.

So how much variation is there?

Summarising the difference in crank velocity during the initial and final pedal strokes:

So the variability in crank velocity for this hard acceleration is greatest in the first few pedal strokes, but then begins to reduce as speed increases, reducing to just +/- 0.2% by the end of the effort.

Impact on power meter accuracy?

Given the size of the crank velocity variance, then it's possible there may be some inaccuracy in reported power. Let's examine one revolution of the crank during the early phase of the sprint to get some idea of what's going on. Here's a zoomed in chart with four lines plotted (click on image to see larger version):

The non-shaded area represents one complete crank revolution - in this case I've chosen the start and end points at the point of peak power (yellow line).

Also plotted is the associated torque profile (red line) in Newton.metres.

Finally, instead of bike speed, I have shown crank velocity in radians per second. There are two speed lines shown, the instantaneous crank rotational velocity (lighter blue line), which varies throughout the pedal stroke, and the average crank velocity (horizontal dark blue dashed line) for that revolution (= 2 x PI / duration of crank revolution in seconds).

Actual Power

Now the true average power in this instance is the average of all the instantaneous power values. In this particular model I am performing calculations for every 1/100th of a second (in some models I use a greater frequency, others less, it depends on the precision needed). A 100Hz "sampling rate" seemed adequate for this purpose.

By averaging all the individual power values, the true average power of the complete crank revolution is 1119W.

What power meters report

However, power meters don't sample crank velocity more than once per crank revolution, they instead they calculate power as the average crank velocity x the average of the torque samples during that crank revolution.

In this case the crank revolution takes 0.770 seconds, which converts to an average crank velocity of 8.16 radians per second. The average of the torque "samples" during that crank revolution was 140.9N.m.

Hence power calculated this way = 8.160rads/s x 140.9N.m = 1146W

That's a difference of 27W, or 2.4%.

Now the variance between maximal and minimal crank velocity during that crank revolution was 4.6%, so we can see that the error in power calculation introduced due to the assumption of constant crank rotational velocity is about half of the maximal variance from the average.

As the sprint progresses, and the crank velocity variation reduces and speed plateaus, then the error in reported power will diminish significantly.

Standing start?

This modelled acceleration was from a rolling start (30km/h), so one might expect greater crank rotational velocity variance in the initial phases of a standing start. But modelling that is for another day.

Something else to keep in mind:

SRM power meters sample torque at 200Hz, hence during this hypothetical crank revolution an SRM would provide 154 individual torque samples, and also uses a magnet triggered electromechanical reed switch to obtain an accurate cadence timing point.

However many other power meter models sample torque at a much lower rate, e.g. 50Hz or 60Hz, meaning there are as few as 38 or 46 torque samples in that example pedal stroke) and some also use an accelerometer divined cadence trigger meaning the exact timing of each pedal stroke may not be as precisely defined and perhaps results in some aliasing of the reported stroke by stroke average crank velocity (cadence), and number of torque samples used.

As a result, power meters that have lower torque sampling rates and that use accelerometer derived cadence may not necessarily report peak power as accurately.